Motion Of Rigid Bodies

What kind of motion can a rigid body have?

Let us try to explore this question by taking some examples of the motion of rigid bodies. Let us begin with a rectangular block sliding down an inclined plane without any sidewise that it does not have translational motion is to fix it along a straight line. The only possible motion of such a rigid body is rotation. The line along which the body is fixed is termed as its axis of rotation. If you look around, you will come across many examples of rotation about an axis, a ceiling fan, a potter’s wheel, a giant wheel in a fair, a merry-go-round and so on


Let us try to understand what rotation is, what characterises rotation. You may notice that in rotation of a rigid body about a fixed axis, every particle of the body moves in acircle, which lies in a plane perpendicular to the axis and has its centre on the axis.


Rotation about a fixed axis
A ceiling fan & A potter’s wheel



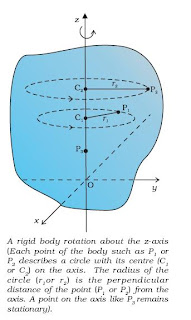shows the rotational motion of a rigid body about a fixed axis (the z-axis of the frame of reference). Let P1 be a particle of the rigid body, arbitrarily chosen and at a distance r1 from fixed axis. The particle P1 describes a circle of radius r1 with its centre C1 on the fixed axis. The circle lies in a plane perpendicular to the axis. The figure also shows another particle P2 of the rigid body, P2 is at a distance r2 from the fixed axis. The particle P2 moves in a circle of radius r2 and with centre C2 on the axis. This circle, too, lies in a plane perpendicular to the axis. Note that the circles described by P1 and P2 may lie in
different planes; both these planes, however, are perpendicular to the fixed axis. For any particle on the axis like P3, r = 0. Any such
particle remains stationary while the body rotates. This is expected since the axis is fixed.

Thus The motion of a rigid body which is not pivoted or fixed in some way is either a pure translation or a combination of translation and rotation. The motion of a rigid body which is pivoted or fixed in some way is rotation.